Twin paradox is real.  Einstein is false.

Home page
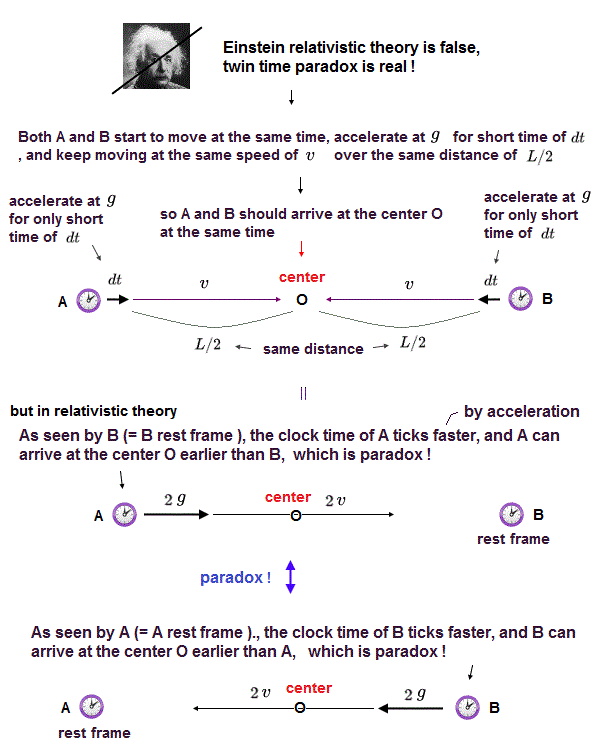
Einstein is wrong
Bell spaceship paradox disproves Einstein

Clock-A sees clock-B moving and running slower.  But as seen by clock-B, clock-A is moving and running slower = twin paradox.

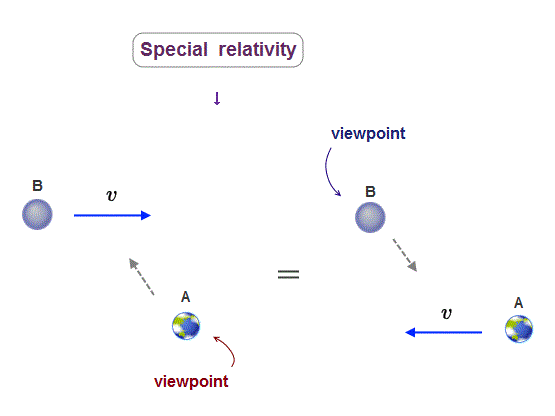
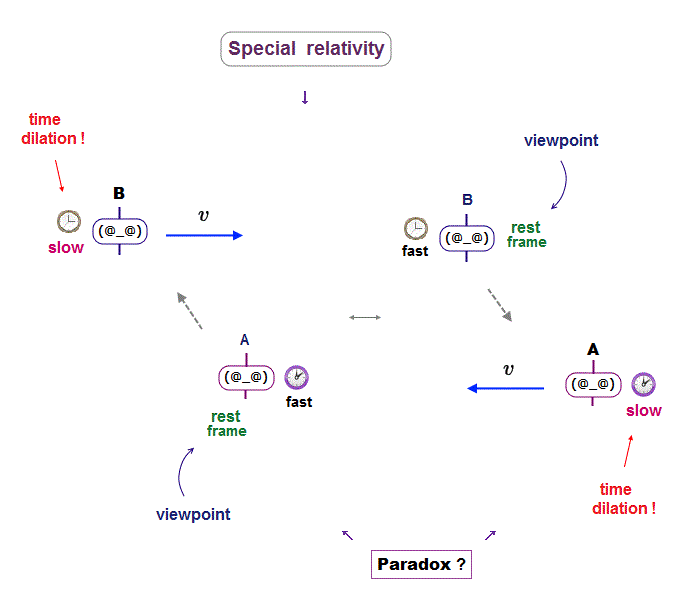
(Fig.1)  Einstein's twin paradox; which clock A or B is moving and running slower is paradoxical and different as seen by different observers.

Einstein relativity says moving clocks tick slower.

Which clock-A or B is moving and running slower is paradoxical, different as seen by different observers A or B.

According to the unphysical Einstein special relativity (= paradoxical Lorentz transformation ), a moving clock is said to tick slower than a stationary clock.  ← illusory pseudo-science

In Fig.1-upper, a clock-A is moving toward a clock-B at rest.  ← The moving clock-A runs slower than the stationary clock-B according to special relativity.

But from the viewpoint of the clock-A , the clock-B appears to be moving toward the clock-A, hence, the clock-B must run slower than the clock-A (= Fig.1-middle ).

↑ This means which clock A or B runs slower is paradoxical and unknown (= called twin paradox ), which shows Einstein relativistic theory is false ( this-p.5-4.conclusion ).

Twin time paradox disproves Einstein.

Einstein relativity is wrong. The paradoxical time dilation is unreal, Not used in GPS

On this page, we show this twin time paradox is a a real fatal paradox, and can Not be solved in either special relativity (= using No acceleration, so just avoiding solving true twin paradox ) or general relativity that unrealistically elongates even rigid objects by fake gravitational (= accelerating ) time dilation.

Contrary to hypes, the paradoxical Einstein relativistic theory is useless, Not applied to any phenomena such as GPS clock time (= relativistic time dilation is too small to measure reliably, this-p.2-3,p.6-conclusion ) and illusory muon's lifetime, as shown in Sagnac effect disproving relativity.

General relativistic time dilation is illusion in gravitational redshift that can be explained by ordinary classical Newtonian energy conservation law.

Neither special nor general relativity can solve twin paradox, so Einstein relativity is wrong.

(Fig.1')  General relativity cannot solve twin paradox, because an accelerating observer must see unreal Rindler black hole and Bell spaceship paradox.

Which clock of twin-A or B runs slower is paradoxical.

A twin-A on the earth sees a twin-B's clock moving by a spaceship and running slower, but the twin-B sees the twin-A's clock moving and running slower ?

As shown in the upper Fig.1', a twin-A remains on the earth from which a twin-B departs, moves in the spacecraft, and returns to the earth.

Seen by the unmoving twin-A's clock on the earth, the moving twin-B's clock runs slower.

But as seen by the twin-B, the twin-A on the earth appears to be moving, so the twin-A's clock must run slower.

↑ Which twin-A's or twin-B's clock runs slower is paradoxical, which is called twin paradox.

General relativity cannot solve twin paradox.

General relativity says only an accelerating twin-B sees unreal ( Rindler ) black hole with negative time which causes unsolvable paradox.

The twin-B in the spacecraft must be accelerating and decelerating to return to the earth, so the frame seen by the twin-B is the "accelerating frame" that needs general relativistic (pseudo-)gravitational time dilation that is said to resolve this twin paradox ( this-2nd-last-paragraph,  this p.3, p,4-(8) ).

Unreal Rindler black hole and Bell spaceship paradox by acceleration

Einstein relativity unrealistically says only an accelerating twin sees pseudo-gravitational field caused by artificial black hole called Rindler horizon ( this-p.4-3rd-paragraph ), which causes new irreparable Bell spaceship paradox unrealistically elongating rigid objects.

As a result, twin paradox is a real, unsolvable paradox ( this-p.1-abstract ) disproving Einstein relativity, contrary to an incredible amount of misleading hyped explanations including youtube ( this-p.3-left-2nd-paragraph ).

Special relativity cannot solve twin paradox.

The clock time seen by the accelerating twin-B needs general relativity, so special relativity alone cannot solve twin paradox, contrary to wrong explanation.

To solve the true twin paradox, we must consider the viewpoint of the accelerating twin-B in spacecraft, which needs general relativity.

But this general relativistic solution needs the unreal black hole called Rindler horizon that causes new irreparable ( time-stopping ) paradox, so can Not solve twin paradox.

So physicists started to ( wrongly ) say the special relativity without acceleration (= called inertial frame ) can resolve the twin paradox.

An illegitimate non-accelerating 3rd-person is used.

In these false special relativistic solutions, they use the 3rd-person moving at the constant speed instead of the accelerating twin-B, which is Not the original twin paradox ( this wrong solution-11~12th-paragraphs use the 3rd-person Albert moving at a constant speed instead of the accelerating twin Sam ).

This typical wrong special relativistic solution used the stationary twin-A's viewpoint (p.6 = Earth's RF ) and the 3rd-person's moving at the constant, non-accelerating speed (p.7 = this RF instead of accelerating Space twin ) who sees the twin-B (= Space twin ) return to the earth at a speed higher than twin-A (= Earth twin ), so the twin-B spacecraft's clock always moving faster ticks slower.

As a result, the special relativity without acceleration also can Not solve twin paradox, and Einstein relativity is proven wrong.

General relativity says a clock in stronger gravitational field runs more slowly, which causes unreal black holes whose time go backward.

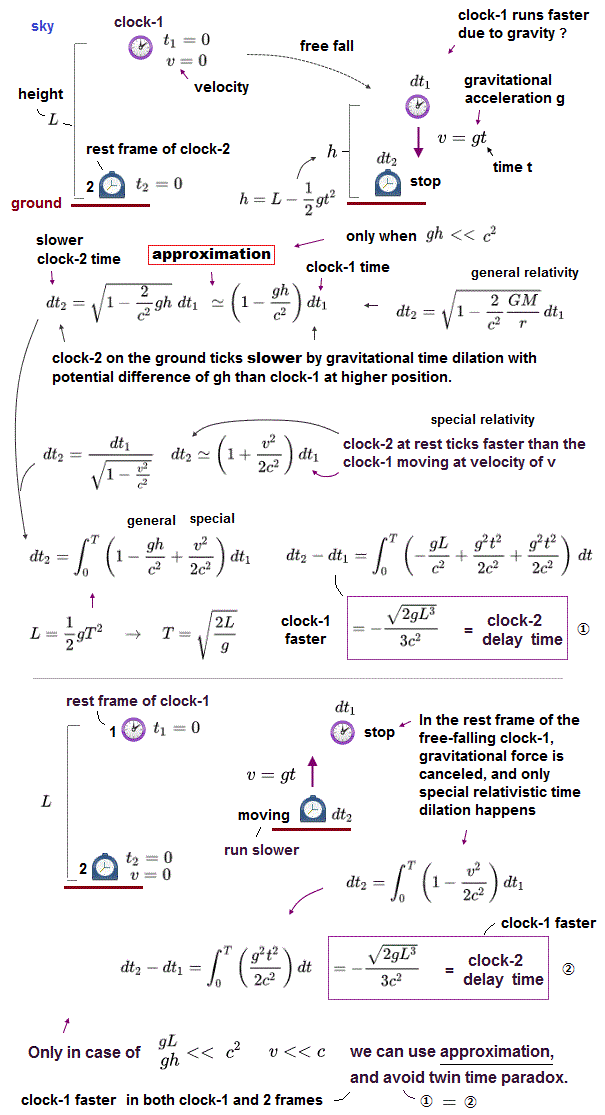
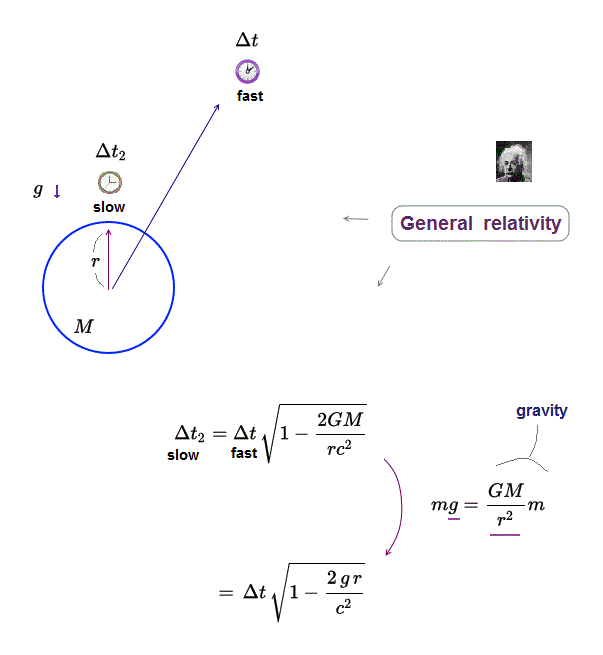
(Fig.2)  Clock-A on the ground runs slower than clock-B at a height of L under gravitational acceleration g according to general relativity.

General relativistic time dilation: clock on the ground ticks slower.

Clocks in stronger gravity (= lower gravitational potential ) run slower in general relativity.

According to general relativity, clock-A on the ground (= closer to a massive star or earth ) ticks slower than clock-B at a height of L under gravity of acceleration g.

This gravitational time dilation is related to the gravitational potential (= φ = -GM/r,  this p.3,p.5 ) equal to acceleration g × the distance (= height ) L = -gL (= Δφ,  this p.3,  this 2nd-last-paragraph ).

↑ Clocks in stronger gravitational fields (= lower gravitational potentials ) tick slower, according to the paradoxical Einstein general relativity.

Gravitational time dilation causes unreal black hole.

General relativity says time in stronger gravitational field (= very lower gravitational potential ) is imaginary or going backward to the past by unreal black holes.

The problem is that this general relativistic gravitational (= acceleration ) time dilation is also paradoxical (= so wrong ), causing unreal imaginary (or negative ) time (= going backward to the past ? ) like inside an unphysical black hole, when the distance L between these two clocks is very long.

Gravitational redshift can be naturally explained by the ordinary classical Newtonian energy conservation law, Not by the paradoxical gravitational time dilation ( this-p.1-right-2nd-paragraph ).

As a result, twin paradox caused by a moving clock ticking slower in special relativity is a real fatal paradox that can Not be fixed by the (paradoxical) general relativity (= gravitational or acceleration time dilation )

As seen by accelerating observers, all things appear to be accelerated by fake gravity which causes time dilation ?

(Fig.2')  As seen by an accelerating clock-A, fake gravitational time dilation happens ?

Einstein says accelerating observers see fake gravity

According to general relativity, accelerating clocks see all things accelerating in the opposite direction by fake gravity, which causes fake gravitational time dilation.

In the upper Fig.2', clock-A is accelerating at g toward a stationary clock-B.

According to Einstein general relativity or equivalence principle, as seen by the accelerating clock-A, all things appear to be accelerating at fake gravity (= acceleration of g ) in the opposite direction, which causes (fake) gravitational (= accelerating ) time dilation related to this fake gravitational potential = gL (= L is distance between clocks A and B,   this-(1)~lower,  this p.5-upper,  this-2nd-last-paragraph,  this p.3-lower~p.4,  this-p.9 ).

Accelerating observers (= frames ) experience fake gravitational time dilation according to paradoxical Einstein relativity.

This-p.2-right-3~p.3-left says
"The accelerated worldline suffers an increasing rate of time dilation, somewhat like gravitational time dilation where increasing height in the gravitational potential results in increasing time dilation"

"an accelerated frame of reference undergoes time dilation similar to gravitational time dilation"

This-p.6-2nd-paragraph says
"That is, clocks at larger z' in the accelerated frame run faster than clocks at smaller z', relative to clocks in the inertial lab frame"

A clock-A, which is moving and accelerating seen by a clock-B, runs slower than the clock-B according to special relativity.

(Fig.3)  Clock-A starts to accelerate at g (= not gravity ) at the distance L from clock-B.  ← This clock-A moving at a speed of v (= gt  g is acceleration, t is time ) ticks slower ?

A moving (= accelerating ) clock-A runs slower ?

As seen by a stationary clock-B, a clock-A is moving and accelerating, so ticking slower in special relativity.

In the upper figure, a clock-A at rest starts to move or accelerate at a acceleration of g at distance L from a clock-B (= this force causing acceleration g is applied only to clock-A in this case, so No gravitational field nor no gravitational time dilation in this frame ), while the clock-B keeps stopping.

The velocity v of this clock-A at a time t is v = gt, which gives the total special relativistic time dilation of the moving clock-A by integrating it with time from t = 0 to t = T where T is the time when the clock-A reaches the clock-B (= figure.3-lower ).

↑ In this case, the moving (= accelerating ) clock-A runs slower than the clock-B at rest according to the special relativity (= no general relativity nor gravitational field is involved in this case ).

Seen by the clock-A accelerating at g, all things including clock-B appear to be accelerating at g in the opposite direction by fake gravity causing artificial gravitational time dilation keeping the clock-A ticking slower ?

(Fig.4)  From the viewpoint of the (accelerating) clock-A, clock-B appears to be accelerating toward the clock-A at fictitious gravitational acceleration of g, which causes pseudo-gravitational time dilation canceling twin paradox ?

An accelerating observer sees fake gravitational time dilation.

General relativity says as seen by an accelerating clock-A, all things including clock-B are accelerating in the opposite direction by fake gravity which causes fake gravitational time dilation.

When the clock-A starts to move and accelerate (= acceleration of g ) at distance of L from clock-B toward the clock-B at rest, from the viewpoint of the clock-A, the clock-B appears to be accelerating toward the clock-A by the fictitious gravitational acceleration of g.

↑ From the viewpoint of the accelerating clock-A, all things including the clock-B appear to be accelerating at g in the opposite direction, which corresponds to the fake gravitational field influencing all things according to (unphysical) Einstein general relativistic equivalence principle.

First, as seen by the clock-A, the clock-B appearing to be moving (= accelerating ) at velocity v = gt (= t is time ) runs slower according to special relativistic time dilation (= upper figure ① ).

Clock-A runs slower due to fake gravity ?

As seen by the accelerating clock-A, the clock-A at the lower fake gravitational potential runs slower than the clock-B in general relativity.

Second, as seen by the (accelerating) clock-A, all things appear to be accelerating at fake gravity (= acceleration of g ), which causes (fake) gravitational (= accelerating ) time dilation related to this fake gravitational potential = gL or gh (= L or h is distance between clocks A and B,   this-(1)~lower ) as shown in the upper figure ②.

↑ In this fake gravity (= acceleration from clock-B to clock-A ) seen by the accelerating clock-A, the clock-A at the lower fake gravitational potential runs slower by the amount of the fake gravitational potential (= - gh/c² ) according to general relativity.

Clock-A always ticks slower than clock-B ?

As seen by clock-A and B, the clock-A always runs slower with no paradox.  ←But the accelerating clock-A sees unreal black hole paradox.

By combining these special (= moving clock-B runs slower in this ① ) and general relativistic time dilation (= by fictitious gravity in this ② ), the clock-A runs slower after all, like the clock-A moving frame (= compare the total time dilation of clock-A of the last of Fig.3 and Fig.4 which are the same.  ← no twin time paradox ? ).

↑ There appears to be no (twin) paradox, because both in clock-A's moving and stationary frames, the clock-A always runs slower by the same amount of time.

Unreal black hole prevents solving twin paradox.

But this fake gravitational time dilation where clocks tick differently in different positions only when seen by the accelerating clock-A causes new irreparable paradox unrealistically elongating even rigid objects and Bell spaceship paradox.

So twin paradox needing an accelerating twin can Not be resolved after all, which disproves Einstein relativity.

Einstein says accelerating observers can unrealistically elongate rigid objects without applying force.

(Fig.5)  An accelerating rigid stick is unrealistically elongated in Einstein relativity.

Einstein relativity cannot conserve energy.

Einstein says an accelerating rigid stick is unrealistically elongated by fake gravitational time dilation, which disproves Relativity.

In the upper Fig.5, a stationary rigid stick starts to accelerate at g maintaining the same length L as seen by a stationary observer K.

Einstein relativity says as seen by an observer K' moving with the accelerating stick, fake gravitational time dilation happens.

In this fake gravitational time dilation seen by the accelerating K', the front of the rigid stick unrealistically elongates due to faster clock time.

And the tail of the stick stops forever due to fake Rindler black hole stopping time (= paradoxically seen only by accelerating observers ).

↑ This rigid stick unrealistically elongated (= impossible ! ) by fake gravitational time dilation shows Einstein is false (= total energy is Not conserved ), and twin paradox cannot be solved.

Feel free to link to this site.