But if we do not fix any values ( for example in the metric "g" ), this theory is completely useless.
So we need to fix this metric to "g" using delta function of
(Eq.1)

where ζ is a gauge parameter.
Top page (correct Bohr model including the two-electron atoms)
Conformal field theory and central charge are physics ?
Superstring theory vs. loop quantum gravity.
In the path integral theory, we have to integrate it with respect to all variables such as X, gauge and metric g (from infinity to infinity).
But if we do not fix any values ( for example in the metric "g" ), this theory is completely useless.
So we need to fix this metric to "g" using delta function of
(Eq.1)

where ζ is a gauge parameter.
ΔFP is caused by the delta function integral of
(Eq.2)

ΔFP is called "FP-determinant".
From Eq.1, we get
(Eq.3)

Sunstituting Eq.3 into the path integral and integrating it with respect to g,
(Eq.4)

From Eq.4, we can choose the mertic g in some gauge ζ we like.
(Eq.5)

Like this page (Eq.4), metric g is supposed to change under gauge transformation as
(Eq.6)

where ω means scale change.
So, fixing the metric of Eq.1 is equal to
(Eq.7)

Metric fixing = variation of Eq.6 is zero = delta function.
Here we introduced the symmetric tensor β (to form scalar), and suppose β satisfies
(Eq.8)

this means β is symmetric and traceless.
Using the following definition of delta function,
(Eq.9)

Using Eq.8 and Eq.9, Eq.7 can be expressed as
(Eq.10)

where β is functional ( which corresponds to p of Eq.9 ), and ω vanishes due to Eq.8.
As shown in Eq.5, we want to know ΔFP instead of its inverse.
To do this, we need to change β and ε to the very strange concepts "Grassmann fields" like
(Eq.11)

Grassmann number is an anti-commuting value like fermions, and satisfies
(Eq.12)

So "integration" is equal to "differentiation" in Grassmann number !
This is strange.
Using Eq.11, Eq.10 becomes
(Eq.13)

where 1/(2π) is chosen to normalize the correlation function.
Even if we change the coefficient of Eq.13, this change is cancelled out.
( coefficients of correlation function × stress-energy tensor. )
See coefficient α of X in this page (Eq.56, 59-61, 73, 74).
Because, Eq.10 can be expressed as
(Eq.14)

And using Eq.12,
(Eq.15)

Eq.14 is the inverse of Eq.15.
As shown in this section, these ghost and Grassmann number are only "mathematical" concepts.
So these are just "ghosts".
Next we change the variables ( x0 = time, x1= space, Euclidean ) into
(Eq.16)

and
(Eq.17)

(Caution: these z and bar-z are different from this page. )
In Eq.17, the boundary condition of space variable x1 becomes 0 = 2π (= circle of complex plane ).
At the time of x0 = -∞, the radius of the complex field circle becomes zero.
At the time of x0 = +∞, the radius of the circle becomes +infinity.
So they take advantage of "mathematical" 2-dimensional string at this point.
According to Noether theorem, when the action is invariant (=symmetry) under some transformation, there are conserved current and charge,
(Eq.18)

where the space is one dimension.
From Eq.18 and boundary condition of x1,
(Eq.19)

so, the total charge Q is conserved.
From this page (Eq.31), this current J is related to stress-energy tensor T, like
(Eq.20)

The space integral means the contour integral of the complex circle, the charge Q becomes (using Eq.18 and Eq.20),
(Eq.21)

The commutation relation between the charge Q of Eq.21 and some primary field φ can be expressed as
(Eq.22)

we suppose Q and φ are time-ordered ( using circle radius ).
Here we use Cauchy's residue theorem of
(Fig.1)

In the residue theorem, only the coefficient of 1/(z-a) is left, like
(Eq.23)
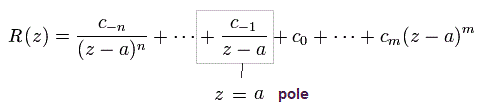 and
and
(Eq.24)
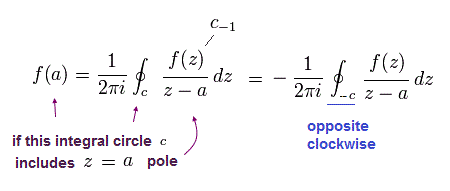
We suppose the OPE of the stress-energy tensor and some primary field φ as
(Eq.25)

The commutation relation of Eq.22 becomes
(Eq.26)

where we use integration by parts in the first term.
So, as shown in this page (Eq.36), we can get the relation of
(Eq.27)

The charge Q proves to be a generator of some infinitesimal transformation.
( But I think they just rely on artificial "mathematical" assumptions here. )
We expand the infinitesimal transformation ε like
(Eq.28)

This is called "Laurent expansion".
From Eq.20 and Eq.21, we define the charge Q as
(Eq.29)

So L become, ( z term )
(Eq.30)

this L is called Virasoro algebla.
Using this page (Eq.74), Fig.1, Eq.25 ( h of tensor T is "2" ) and Eq.30, we have
(Eq.31)

where we use the integration by parts and residue theorem.
Finally, we get
(Eq.32)

If "c" of Eq.32 is not zero, Eq.32 is not beautiful for symmetry and group theory.
So they insist the central charge of Eq.32 needs to be zero.
( And if c is not zero, generator rule of Eq.25-Eq.27 is violated, when φ of Eq.27 is T. )
Instead, strange 26 dimensions (or 10 dimensions) have to be accepted !
Unfortunately, these are only "math", and not real.
( Which is more important, extradimensions or symmetry, you think ? )
From this page (Eq.56, Eq.83), the total action (= scalar field + ghost ) becomes
(Eq.33)

where we introduce bar-X.
If we suppose these variables change like
(Eq.34)

this is called "BRST transformation".
The action Eq.33 is invariant under Eq.34, like
(Eq.35)

where we use
(Eq.36)

In this way, it seems that they are trying to make new "mathematical" transformation and symmetry.
But the most important thing is whether these symmetries are actually linked to this real world or not.

2012/8/22 updated. Feel free to link to this site.