
Home page
Calculation of QED g-factor.
Einstein relativity is false.
(Fig.1) A electron's bare charge and mass must be infinite to cancel QED meaningless infinities and artificially get finite g-2 (= anomalous magnetic moment ) values.

(Fig.2) QED renormalization just illegitimately removes infinities.
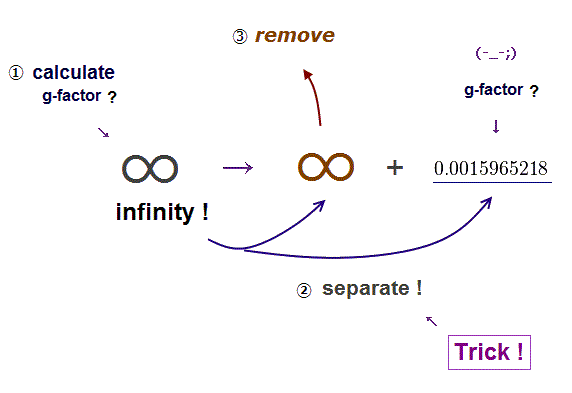
Contrary to the media-hype, quantum electrodynamics (= QED ) is an unsuccessful, unreal, useless theory.
Relativistic QED says the vacuum is filled with unphysical virtual photons with imaginary mass, which paradoxically disobey Einstein relativistic mass-energy relation (= called off-mass-shell ), to mediate electromagnetic force ( this-p.3, this-p.16 ).
These infinite (unreal) virtual particles make all QED calculations give meaningless infinities ( this-3rd-paragraph, this-3rd-paragraph ).
↑ These QED meaningless infinities must be artificially removed by the illegitimate math trick called renormalization redefining bare mass-charge free parameters to get some finite values of electron's anomalous magnetic moment (= g-2-factor ) and Lamb shift.
This QED renormalization artificially removing infinities to illegitimately get some desired finite values is harshly criticized even by founders such as Dirac and Feynman as "hocus-pocus" or "dippy process ( this-A. Historical introduction, this-1st-paragraph )".
This-p.1-right-2.2~p.2-left says
"Unfortunately, QFT (= quantum field theory or QED ) was a complete failure since any attempted calculation under this theory resulted in an (meaningless) infinite number"
"Separation: separating an infinite series into two components, one that diverges to infinity and another that converges to a finite value. Eventually, the infinite component is ignored and only the finite part (= anomalous magnetic moment ) remains" ← QED is a wrong math.
This-1st-paragraph says
"trick of theoretical physicists in sweeping infinities under the rug using what is called renormalization. Roughly speaking, renormalization solves the infinity problem by adding a negative (arbitrary) infinity to the positive infinity to cancel it out" ← Not a QED prediction.
This-p.3 (or p.1-introduction)-2nd-paragraph says
"if we would be able to arrange the infinities of the bare quantities and the
infinities coming from the divergent integrals to cancel each other systematically,
we would be left with a finite (= artificial trick )"
According to the unphysical QED, the bare charge and mass of an electron must be unrealistically infinite to cancel the infinities caused by infinite virtual particles ( this-p.3-5, this-middle, this-p.19-3rd-paragraph, this-middle, this-p.53 ).
This QED's illegitimate removal of infinities by artificially redefining fictitious mass and change is call "mass or charge renormalization ( this-p.2 )".
This-3rd-4th-paragraphs say
"Renormalisation refers to a term's ( artificial ) redefinition based on mathematical properties, which help eliminate infinity. A fundamental concept here is separating infinite from measurable finite physical variables. It is done by adding counter terms to the original Lagrangian of a given theory."
"The electron’s observed mass and charge are not the "bare" mass and charge in the original Lagrangian. They are "renormalised" values that take into account quantum corrections (= free parameters, so Not QED prediction ). The renormalisation method modifies these constants to fit experimental results, hence removing the infinite values."
This-p.18-last says
"In analogy
to the mass renormalization, this infinity is absorbed into a redefinition (= artificial manipulation )
of the electric charge — charge renormalization ( this-p.2 )"
This QED math based on infinity minus another infinity giving some finite physical values is clearly wrong and illegitimate.
↑ According to legitimate math, "infinity minus infinity" becomes a "uncertain indeterminate number" that cannot determine some physical values, so QED renormalization artificially removing infinities is Not a right math.
Not only the electron's anomalous magnetic moment but also tiny Lamb shift in QED is also an artificial illegitimate manipulation irrelevant to QED prediction as
this-p.3-left-6th-paragraph says
" His calculation (= of Lamb shift ) included this value that
we suspect that was entered ad hoc to match the theoretical
value with the experimental value. In the field of physics, this
trick is known as a 'fudge factor'."
If QED was really successful or useful, almost everybody should have already known how to calculate the anomalous magnetic moment by such a (wrongly) successful QED. ← But actually, ordinary people (even physicists) do Not know any QED (meaningless) calculation methods based on (unreal) virtual particles ( this-p.5-right-1st-paragraph, p.6-(22) ), which fact shows QED is useless, unreal.
(Fig.3) QED unreal virtual electrons and photons are expressed as unphysical math relations called propagators that are used as a tool to artificially manipulate anomalous magnetic moment value.
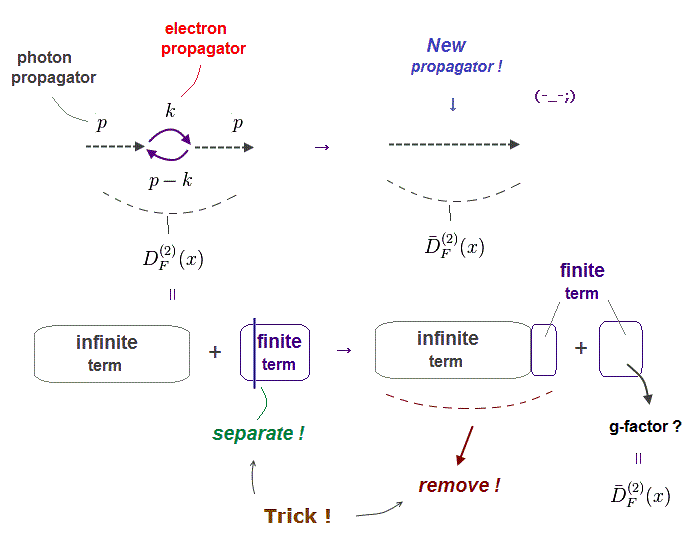
The (unphysical) QED is rather simple, consisting only of illusory virtual electrons and photons with energies (or momentums ) from minus infinity to plus infinity, which QED unphysical calculations always diverge to meaningless infinities by integration ( this-p.8-upper, this-p.68(or p.64), this-p.6 ).
In the unphysical QED rule based on abstract Feynman diagram relating (illusory) photons to electrons, these virtual electrons (= SF ) and virtual photons (= DF ) are expressed as unphysical math relations called propagators of (illusory, virtual) photons ( this-p.2-4, this-p.2-3 ) and fermions (= virtual electrons, this-p.9-10, this-p.2 ).
Here "p" represents a (virtual) particle's relativistic momentum and energy, κ represents the electron's mass, λ represents the photon's mass (= this λ should be close to zero ).
These virtual photons and electrons are known to disobey Einstein relativistic energy-mass relation ( this-p.9-10, this p.6 ), disproving relativistic QED,
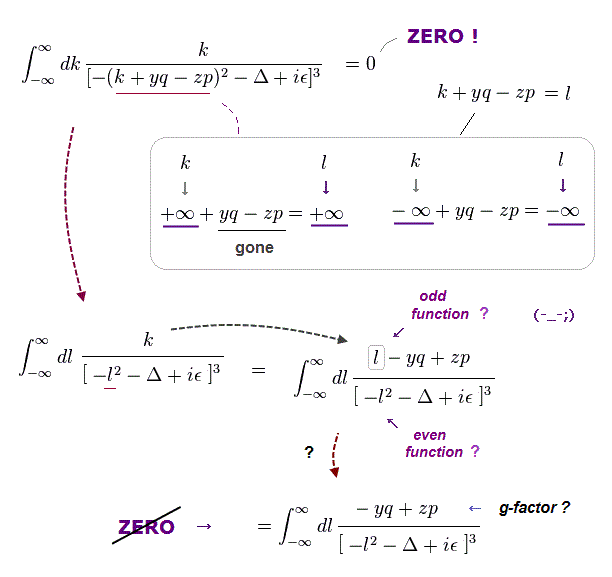
(Fig.4) QED just artificially changes equations and removes the unneeded part by illegitimate renormalization to get finite anomalous magnetic moment.
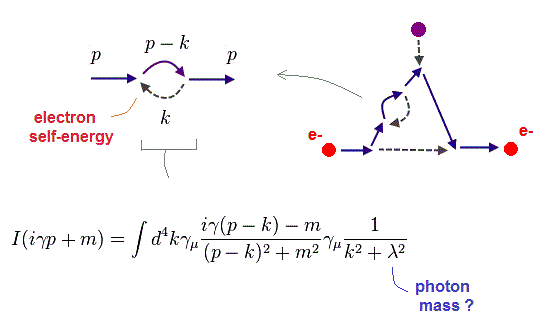
Fig.4 is part of electron's anomalous magnetic moment (= g-factor ) allegedly caused by the unreal virtual photons and electrons ( this p.9-10, this-p.6-8 ) in the two-loop or fourth-order correction calculated by Karplus and Kroll in 1950 ( this p.24, this-p.10-11 ).
The first calculation (of one loop correction of anomalous magnetic moment ) by Schwinger no longer agreed with the new experimental result, so Karplus and Kroll tried to artificially fit the newly-calculated two-loop correction (= fourth-order ) of anomalous magnetic moment with the new experimental value.
↑ Later, after the newer experimental result of the anomalous magnetic moment turned out to disagree also with the new Karplus, Kroll's calculated results, they started to say their (artificial) calculations of Fig.5 might be wrong (= Fig.4 calculation remained as a right one ).
↑ This infamous unscientific story showed QED is just an illegitimate math trick artificially fitting calculations with new experimental results ( this-p.5-right-6.1~ p.6 ), each time the previous artificial QED calculated values turned out to disagree with the new experimental values.
This Karplus and Kroll's paper ( p.2-Fig.1-IId, p.5-right~lower,p.7, p.10-MIId, p.12,14-μIId ) explains the detail of Fig.4's calculation of part of anomalous magnetic moment based on (unreal) virtual photons and electrons.
In this artificial calculation process of the anomalous magnetic moment's two-loop correction, they deliberately used a wrong math in calculating this-p.6-equations (21) and (22) (= this (21) is Not equal to (22) as shown in Fig.4-artificial illegitimate change, contrary to the paper's claim, without some unspecified artificial new rules ).
↑ After this wrong math illegitimately changing the equation, only one term of this artificial equation-(22) in p.6 (= Fig.4's equation surrounded by the square ) was used as part of anomalous magnetic moment in MIId in this-p.10, and all other parts (= including divergent and non-divergent parts ) were artificially removed by the illegitimate renormalization based on illusory infinite bare charge or mass.
↑ This-p.7-left-2nd-paragraph (of this two-loop anomalous magnetic moment calculation ) says
"The fact that B is infinite,.. it can be interpreted as a charge renormalization (= the first part of I was artificially removed by using the unreal infinite bare charge )"
This-p.6-(22) says
"The first term... and is therefore canceled by the (illegitimate) mass renormalization"
↑ As a result, QED artificially removed only the unneeded infinite and finite terms by illegitimate renormalization based on unreal infinite bare mass and charge to leave only the part surrounded by the square ( compare this-p.6-(22)(23) and p.10-lower-MIId ), which is Not a prediction.
QED is an unsuccessful illegitimate math trick unable to predict the anomalous magnetic moment (and Lamb shift ), contrary to hypes.
(Fig.5) QED math trick: artificial change of variables → remove only the unneeded part by renormalization, which is Not prediction.
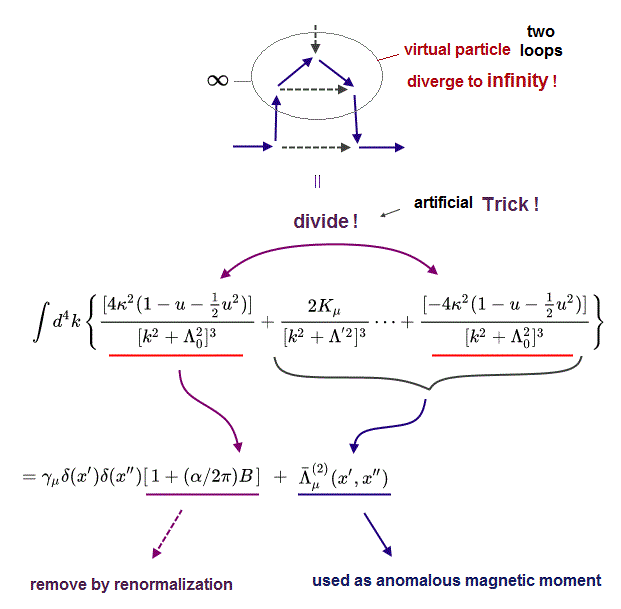
Fig.5 is QED artificial math trick in another part of electron's anomalous magnetic moment's two-loop correction ( this-p.2-Fig.1-IIc, p.8, p.9-upper, p.10-MIIc, p.14-μIIc ).
This-p.8-(29) conducted the artificial change of variables, and then separated, removed only the unneeded part of B in the final anomalous magnetic moment part of MIIc in p.10.
This-p.9-2nd-paragraph says
"Here again the separation of the renormalization term B has made the physically significant correction divergent ( this-p.2-3rd-paragraph )"
↑ This Karplus, Kroll (artificial) calculation of electron's anomalous magnetic moment's two-loop (= fourth-order ) correction almost agreed with the experiment at first.
But later, after a newer experimental result turned out to disagree with this Karplus, Kroll's calculation, they started to say there might be some calculation mistake, and artificially changed (= manipulated ) the calculated result, which is illegitimate, Not a QED prediction at all ( this-p.5-6.1-p.6 ).
After Petermann's new (unpublished) calculation, Fig.5 calculated result (= μIIc ) was artificially changed, and Fig.4 result (= μIId based on wrong math disproving QED's anomalous magnetic moment ) remained, unchanged ( compare this-p.2 and this-p.14 ).
This unphysical too-loop correction artificially leaves the terms proportional to γμ or γν as part of anomalous magnetic moment (= new artificial L surrounded by the square, this-p.8-(32), p.9-(33), p.10-MIIc includes γμ (= γμ ) or γν terms as part of anomalous magnetic moment ), which ad-hoc new rule is inconsistent with the one-loop correction removing all γμ terms.
↑ It means QED just artificially separating and removing the unneeded part by illegitimate renormalization is unable to predict any physical values such as anomalous magnetic moment.
(Fig.6) QED depends on illegitimate change of variables and renormalization of infinities to artificially generate some finite physical values.
Contrary to the hypes, QED calculation is unsuccessful, wrong, giving only meaningless infinities.
QED often uses the artificial math trick of change of variables.
↑ "Change of variables" itself originally should not change the results themselves, which is Not the case of QED that artificially separates and removes the unneeded part artificially.
In order to obtain the desired anomalous magnetic moment value, QED artificially separates and removes only the unneeded part by the illegitimate renormalization ( this-p.6-1st-paragraph, p.7-left-2nd-paragraph, p.8-(29). p.9, this-p.26-A.28 ).
So by combining the change of variables with the illegitimate renormalization, QED can artificially manipulate the finite results as free parameters, which shows QED is unsuccessful with No ability to predict any physical values.
This-p.9-last-MI alone has the unknown additional coefficent of π2.
This-p.8-last-(-α/2π) of L is artificially eliminated in this-p.9,p.10-MIIa.
This-p.4-right-footnote says different physicists used different definition or different coefficient values, which is Not QED prediction.
This-p.12-(56)(57), p.14 shows all infinite terms of 1/2lnλ2/κ2 (= photon mass λ → 0 ) have unnaturally the same simple coefficeint of 1/2 to artificial cancel each other's infinities, though other coefficients are complicated and different values.
Even after the alleged correction of calculation mistake ( this-p.2-μIIc ), only the infinity part of 1/2log λ2/m2 had the unchanged simple coefficient 1/2, while other coefficients changed. ← This is unnatural.
↑ This shows they artificially adjusted and separated the original infinity into the infinity (= the same 1/2lnλ2/κ2 to calcel each other ) and finite parts (= infinite part can absorb arbitrary unneeded finite numbers, which is artificial manipulation of anomalous magnetic moment ), which is Not prediction.
(Fig.7) QED artificial change of variable and renormalization removing infinity is the illegitimate math trick irrelevant to real physical phenomena.

Another two loop QED correction of anomalous magnetic moment used the artificial illegitimate math trick.
Fig.7 case is part of anomalous magnetic moment originating from the unreal virtual photon (= expressed just as unphysical math propagator ) splits into two (unreal) virtual electrons-3 and 4 ( this-p.2-right-Fig.1-IIe. p.4-second-order functions, p.5-(13), p.9-MIIe, p.10-MIIe, p.11,p.14-MIIe, μIIe ).
To obtain the unphysical equation of this-p.4-(12), they used artificial change of variables and transformation that is irrelevant to prediction of real physical phenomena.
↑ This-paper explained this artificial transformation expressing virtual electrons-3,4's energies and moments as k' k'', virtual photon as k. ↓
This-p.25-(A.13)(A.14)-p.26-(A.27)(A.28)-artificial change of variables and transformation, (A.32)-artificial partial integration with respect to artificial new variable of v, they artificially separated infinite divergent term from finite terms artificially created by the change of variables into v. p.27-(A.35).
This-p.5-left-(13) says "This infinite constant, however, has no observable consequence", which unneeded part was illegitimately removed by renormalization.
Compare this-p.4-(12) and p.10-last-MIIe where the unneeded infinite or finite parts were artificially removed by illegitimate renormalization.
As a result, QED just manipulating anomalous magnetic moment value by illegitimate change of variables ( this-p.26-(A.28) ) and renormalization ( this-p.5-(13) ) can Not predict any physical values, contrary to hypes.
QED for all other atomic g-factor or magnetic moments (= like the free electron's magnetic moment ) needs to artificially choose fake effective potential energy V, basis set functions, and adjust free parameters, which can Not predct magnetic moments, contrary to hypes.
↑ This-p.2-S1-effective (= fake ) magnetic interaction Vg, p.5-last-paragraph-free-parameter-λ, p.6-3~4th-paragraphs say select artificial CH potential, this-p.6-artificially-chosen-potentials based on DFT. this-p.18-lower-artificially-chosen parameter ξ this-p.4-right-artificially-chosen-screening potential approximation. ← No QED prediction
The fictional muon's anomalous magnetic moment or g-2 factor is calculated in the same way as QED in the artificial electron's anomalous magnetic moment of removing infinities by renormalization.
This QED prediction is known to disagree with the muon's g-2 anomalous magnetic moment which can not be measured directly.
So physicists started to rely on ad-hoc lattice QCD (= quantum chromodynamics ), which is completely different from QED method (= so QED failed ), to explain the discrepancy between today's QED standard model's prediction and the measured muon's g-2 magnetic moment ( this-middle-lower, this-lower, this-9th-paragraph ).
↑ Contrary to many hypes such as "(wrongly) successful QED or QCD", this lattice QCD is unable to predict any physical values, because QCD must rely on artificial models and many freely-adjustable parameters.
↑ This latest paper on muon's g-2 ↓
p.30-2.3.5. Lattice QCD approach-3rd-paragraph says
"starting from a model of the charged and neutral pion form factor,.. one could fit their (squared) difference to the lattice correlators to fix a few of their free parameters"
p.31-Table 2 says "the second errors due to the difference between the (artificially-chosen) GS and KS models... systematic uncertainty arising from using different dispersive parameterizations"
This-p.62(or p.41 )-last-paragraph also says "The GS model has two free parameters"
The 6th paragraph of this hyped news (4/28/2025) says
"Measurements on the stable isotope Bi-209 were recently consistent with the theoretical prediction. However, there were still doubts as to whether the influence of the nuclear structure on the theoretical prediction "
↑ This research paper's abstract says
"Our result for the hyperfine splitting (= related to nuclear spin magnetic moment that cannot be predicted by QED nor QCD ) is in excellent agreement with the most accurate prediction based on a combination of quantum electrodynamics calculations with an empirical (= so Not theoretical prediction ) treatment of the hyperfine-structure"
↑ This research paper-p.39(or p.27)-last-paragraph says
"Currently, it is still Not possible to derive the magnetization distribution from first principles.
Therefore, various models are used to describe it" ← QED or quantum mechanics can Not predict nuclear values, either.
In all QED calculations of multi-electron atoms, physicists have to artificially choose (fake) wavefunctions (= finite basis sets, this-p.2-3 ), ad-hoc (fake) screening potential ( this-p.2-2nd-paragraph ) of inner electrons and various nuclear models ( this-p.1 ), which can Not predict any physical values.

Feel free to link to this site.