Home page
Quantum computer is useless
QuEra's error-prone magic state
Neutral atoms are fake simulators.
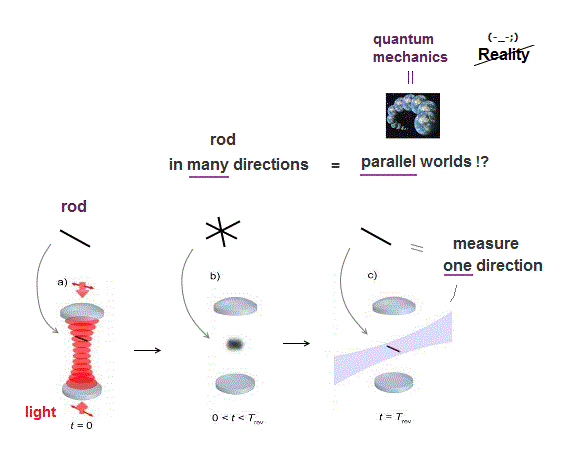
(Fig.1) Practical control of fragile neutral atomic qubits unstably trapped in laser light is impossible. ← Practical quantum computer is impossible.

Contrary to an incredible amount of hypes, neutral atomic qubits (= each atom's two energy levels are used as one qubit's 0 or 1 states ) unstably trapped in laser light lattice (= optical tweezers ) at cold temperature is useless for quantum computers.
It is impossible to control the positions and numbers of atoms (= atomic qubits ) trapped in laser light, which is too unreliable to use for practical quantum computers.
Atoms easily get lost and dissapper from the laser light, so physicists need to constantly load new atoms onto laser light randomly with the loading probability of just 50% ( this p.1-right, this p.1-left-2nd-paragraph ).
This-Neutral atom quantum computers-4th-paragraph (6/4/2025) says
"However, trapping and stabilizing neutral atoms is challenging because minor misalignments or power changes in the laser beams can cause atoms to drift or escape"
This-Core qubit technologies-Neutral atoms (4/16/2025) says
"In this technology, a qubit is encoded in two hyperfine ground-state levels of an atom... but its challenges center on scaling to a million qubits, control electronics, and error rates, which are not yet clearly possible"
This 6~7ths-paragraphs say
"loading the atoms into the tweezers is a stochastic process—which means that the probability of each trap being occupied is 50%" ← error rate of 50% !
This 13th-paragraph says
"The time that the atoms stay in the optical tweezer is not that long. It is typically only a few milliseconds, and it is limited by the rate at which additional atoms are loaded into the trap." ← very unstable atomic qubits
This p.5-left-1st-paragraph says
"The result indicates that the probability of losing
a single atom across the entire array remains under 50%
during 100 ms" ← The error rate of 50% (= due to loss of atoms ) per 100 ms was too high !
Even by repeatedly loading new atoms onto optical lattice, the total number and positions of the loaded atoms are uncertain and fluctuating ( this p.5-Fig.4 ), which cannot be used for making reliable precise quantum computers.
The 5th paragraph of this hyped news (3/3/2025) says
"The team's method, detailed in a recent paper in PRX Quantum, achieved 93.4% accuracy (= still too high error rate ) in detecting lost atoms (~ just detect lost atoms, Not filling it )"
↑ This-paper-p.9-Fig.5 shows this neutral atomic qubit's survival probability drastically decreased to 0% in only 40μs (= neutral atomic qubits are too short-lived to be practical ).
This recent hyped news (9/22/2025) says 3000 neutral atoms (= 3000 qubits ) could barely be maintained for just 2 hours (= so this quantum computer is broken just 2 hours, useless ) by constantly reloading new atoms. ← Each qubit's state's error rate was too bad
↑ As shown in this-p.19-extended data fiture 6-a,c, the number of total atomic qubits and their positions were unstably fluctuating (= constantly causing errors ). ← Precisely control of each atomic qubit for some computation is impossible due to high error rates in these easily-lost atomic qubits.
Controlling and reading atomic qubits are impractical, taking much more time than other types of qubits such as superconducting qubits.
The 6th-paragraph of this site (12/4/2024) says
"engineers are still working to improve the accuracy (fidelity = still error-prone neutral atomic qubit ) and speed of their two-qubit operations (= too slow )."
This-lower-disadvantage of neutral atom qubits (3/28/2025) says
"Difficult to Manipulate: Precise control of neutral atoms, especially when scaling to larger systems, remains a significant experimental challenge.
"Slow Gate Operations: The manipulation of neutral atoms requires slower processes compared to other qubit types, limiting the speed of quantum computations."
This 16~17th paragraphs say
"One reason the neutral-atom qubit isn't the front-runner in the quantum computer race is speed.... more than 1,000 times slower than a superconducting-qubit system..... they are finicky to operate.... researchers have yet to work out how to efficiently and rapidly operate more than a handful of neutral-atom qubits"
Just reading each atomic qubit 0 or 1 state by illuminating it with light is impractically slow and taking much more time (= ~ 80ms, this p.3-right-last-paragraph ).
So this neutral atomic qubit (= using excited Rydberg atomic state ) is about 10000 times slower than superconducting qubits (or classical computers ) used by IBM, Google.
↑ This Platform table shows superconducting qubit's speed 1.4 MHz is 10000 times faster than neutral atomic qubit (= Rydberg arrays )'s 170 Hz (= too slow to be practical ).
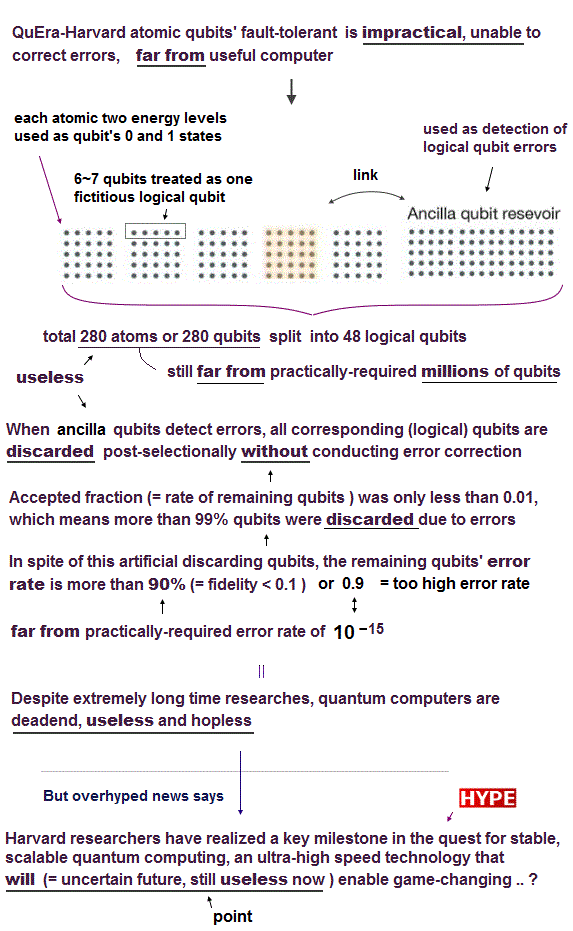
(Fig.2) Harvard-QuEra used only 280 unstable atoms divided into 4 or 48 (logical) qubits (= just 4 or 48 bitstring ) with extremely high error rates (= more than 90% error ) and No ability to correct errors. ← practical quantum computer is hopeless
Contrary to the overhyped news like "Key step toward reliable, game-changing quantum computing", Harvard-QuEra recent research published in Nature can never be a practical quantum computer.
They used only less than 280 cold atoms unstably trapped in laser light ( this 5th-paragraph ) as quantum bits or qubits: each atomic two energy levels were used as a (qu)bit's state 0 or 1 whose bit states could be changed by laser light ( this-middle neutral atom ).
But today's quantum computers are useless, too error-prone to give right answers.
They cannot use their 280 atoms as 280 qubits (= each qubit takes 0 or 1 states ), instead, they had to split 280 atomic (physical) qubits into just 4 or 48 (fictitious) logical qubits.
↑ So this Harvard-QuEra used only 4 or 48 bitstring (= each logical qubit can take only 0 or 1 state ), which is still Not a computer.
↑ Far from millions of qubits needed for a practical quantum computer ( this p.1-left-1st-paragraph ).
The point is today's quantum computers are too error-prone to correct their errors, because the operation of error correction itself increases the original errors instead of decreasing errors.
So physicists have No choice but to rely on illegitimate cheating called "post-selection" which just discards erroneous results or qubits without error correction.
↑ This Harvard's illegitimate post-selection approach cannot scale up quantum computers, because today's error-prone qubits so easily cause errors that almost all qubits must be discarded due to showing errors, which discarded qubits cannot be used for final calculated results.
This 5~6th-paragraphs say
"280 of these atoms were converted into qubits and entangled with the help of additional lasers, which resulted in the creation of 48 logical qubits."
"Instead of fixing mistakes that occur during computations (= instead of correcting errors ), the processor used by the Harvard team incorporates a post-processing error-detection phase. During this phase, erroneous outputs are discovered and discarded (= without error correction )"
The 4th-last paragraph of this site says
"What the Harvard team’s processor does, rather than correct errors during calculations, is add a post-processing error-detection phase wherein erroneous results are identified and rejected." ← illegitimately post-selection without error correction.
This 17-18th, 21th paragraphs say
"This isn't full error correction. What is happening in the paper is that the errors are corrected only after the calculation is done (= post-selection of results luckily avoiding errors )"
"But as the researchers got more stringent about rejecting measurements with indications of errors, the results got progressively cleaner. One measurement of accuracy rose from 0.16 to 0.62 (= still 84~38% error rates, even after discarding or rejecting erroneous results post-selectively.)"
"this isn't full error correction done while calculations are in progress, and QuEra is working on that. In addition, the algorithms used in these tests aren't useful in the sense that no commercial customer would pay to run them" ← useless and unable to correct errors.
This 33th, 35th paragraphs say
"they can preferentially reject measurement outcomes with errors, and therefore identify a subset of outcomes with lower errors. This approach is called post-selection, and while it can play a role in quantum error correction, it doesn't by itself solve the problem."
"Though there's still more to be done (← still useless )"
(Fig.3) Harvard-QuEra atomic qubits are too error-prone, useless, unable to correct errors.

↑ This research paper ↓
p.2-left-2nd~3rd-paragraphs say
"This architecture is implemented using
arrays of individual 87Rb atoms trapped in optical tweezers,"
"We use systems of up to
280 atomic qubits" ← up to 280 Rb atoms trapped in laser light were used as 280 qubits.
p.4-Fig.3 shows each logical qubit consists of 7 physical qubit (= Fig.3a ), and they tried to prepare 4 logical qubit's GHZ state = 4 qubits are all in the 0000 or 1111 states (= Fig.3e ).
↑ GHZ fidelity without postselecting on flags (= nFT = raw results ) was very bad = only 0.55 (= Fig.3c, nFT ), which means 45% error rates just for making 4 qubits the simple 0000 or 1111 bitstring (= GHZ ) states .
p.4-left-last-paragraph says "in which syndrome events (= ancilla qubits detecting errors ) most likely to have caused algorithmic failure are discarded (= discarding all results where ancilla qubits showed errors ).."
".. for example, discarding just 50% of the data improves GHZ fidelity to approximately 90%" ← Discarding 50% qubits or results (= accepted fraction was 0.5 ) due to showing errors without error correction improved GHZ fidelity to 0.9, as shown in Fig.3d or this Fig.
p.5-right-4th-paragraph says "To characterize the distribution overlap, we
use the cross-entropy benchmark (XEB)...
XEB = 1 corresponds to perfectly
reproducing the ideal distribution (= XEB = 1 is errorless or fidelity = 1 ) and XEB = 0 corresponds to the
uniform distribution, which occurs when circuits are overwhelmed by
noise (= XEB = 0 means 100% error rate or 0% fidelity )."
↑ XEB is equal to fidelity, so XEB = 0.35 means error rate of 65% ( this 10th-paragraph ).
p.5-right-5th-paragraph says "We obtain an XEB of approximately 0.1 for 48 logical qubits" ← 48 logical qubits showed 90% error rate (= XEB or fidelity was 0.1 ).
p.6-Fig.5e shows 48 logical qubits managed to get 0.1 XEB fidelity (= 90% error rate ) when the accepted fraction was less than 0.001 (= 90% error rate even after 99.9 % of the results or qubits were discarded due to showing errors ).
↑ These atomic qubits were useless, too error-prone (= actual error rate was more than 99.9% ), unable to correct errors (= instead, they just discarded or ignored erroneous results ).
↑ So even after 99.9% qubits or results were discarded postselectively without error correction, the remaining qubits showed 90% error rates, which is completely useless.
The 13-14th of this blog says
"With their IQP demonstration, they needed to postselect on the event that no errors occurred (!!), which happened about 0.1% of the time with their largest circuits (= which means 48 logical qubits showed 99.9% error rate, which must be discarded post-selectively )"
"They don't claim to have demonstrated quantum supremacy with their logical qubits—i.e., nothing that’s too hard to simulate using a classical computer." ← Quantum supremacy was fake.
Today's quantum error correction manipulating qubits is useless just increasing and worsening errors.
So all they can do is virtual error correction using classical computer's software without directly correcting erroneous qubits, as shown in this p.3-right-1st-paragraph says
"we can detect the presence of physical qubit
errors, decode (infer what error occurred) and correct the error simply
by applying a software ZL/XL correction24"
↑ This reference 24 paper's p.17-right-IX says
"The logical operators XˆL and ZˆL
that we have spent so much time discussing are Not actually implemented in the surface code hardware (= Not qubit hardware error correction )! These
operations are handled entirely by the classical control
software" ← No quantum error correction after all.
(Fig.4) Quantum simulator is fake, useless, unable to really simulate or calculate physical phenomena.
Physicists often pretend that neutral atoms trapped in optical lattice (= laser light ) are (imaginary) electrons of solid crystals as fake quantum simulators that is useless, unable to really simulate or calculate any physical phenomena.
This-p.2-right-2nd-paragraph says
"we understand that an
optical lattice acts as an artificial crystal (= optical laser = fake crystal !? ) created by
laser lights, and confined atoms behave just like electrons in solids (= neutral atoms = fake electrons !? )"
This-5th-paragraph says
"the system of neutral atoms in the optical lattice simulates a real material (= which does Not mean the ordinary computer simulation, because the error-prone unstable neutral atomic qubits can Not simulate nor compute anything ): the neutral atoms behave as electrons (= which does Not mean neutral atomic qubits computed nor really simulated electrons' behavior ) and the optical lattice mimics the periodic crystal structure"
The 2nd, 6th paragraph of this hyped news (6/4/2025) say
"String breaking occurs when the string between two strongly bound particles, such as a ( unseen fictional ) quark-antiquark pair, breaks and new particles are created."
"The van der Waals interactions between the Rydberg atoms used here imply that two atoms cannot be excited at the same time if they come very close to each other. They block each other,... This effect reflects the restriction that elementary particles such as gluons or quarks face due to the strong interaction"
↑ So this fake quantum simulator tried to associate the van der Waals interaction between neutral atoms with the irrelevant (fictional) quarks' strong force.
Even the hyped news (= this-middle, the noisy intermadiate-scale quantum era ) says (7/9/2025)
"The second challenge is that quantum logic gates have low fidelity. Over a long sequence of operations, errors accumulate, corrupting the result."
"Finally, quantum simulators currently have a very limited number of qubits – typically only a few hundred. This is far fewer than what is needed for high-energy physics (HEP) problems." ← Today's useless quantum computers cannot simulate anything such as quarks.
↑ This-paper ↓
p.2-Fig.1-(d) says "String breaking in the Rydberg system, where particle-pairs are created by flipping the state of one atom." ← They tried to treat two neutral ( Rydberg ) atomic energy state change as the imaginary unseen quarks' strong force breaking in nuclei (= p.1-left ).
p.6-right-Discussion and outlook says
"the present observations are limited by imperfections in many-body
state preparation, by Rydberg blockade violations and by
decoherence arising from atomic thermal motion, decay
and dephasing"
p.10-left says 256 atoms. ← just 256 atomic qubits (= one atomic qubit can take only 0 or 1 value ) is still Not a quantum computer.

Feel free to link to this site.